Coherent Edge Radiation
Edge Radiation
In a bend relativistic, charged particles emit synchrotron radiation with the characteristic opening angle of 1/gamma and the characteristic wavenumber kc=gamma3/R, where R is the bend radius. The characteristic wavenumber is a rough estimated, for which the emission is maximized. Beyond the emission quickly falls off for higher wavenumbers.
However the spectrum and the angular distribution is altered in the transient regime of the bend dipole. The typical footprint is very similar to transition radiation and it is called "Edge Radiation" (ER). The key parameter is the formation length of the radiation, which is given by Lf = lambda / (1-betaz), where betaz is the normalized velocity in the direction of observation (for convenience we oriented the z-axis in that direction). Note that within the bend the formation length is analogous to the characteristic wavenumber. Longer wavelength would require a longer propagation in the direction of observation for a better emission, thus the suppression in the emission of synchrotron radiation towards longer wavelength.
To understand the source of edge radiation we look closely to the motion of the electron in a bending system with respect to a fixed observation direction. The transverse acceleration is the driving source for the synchrotron like radiation with the field polarization in the direction of acceleration. In the longitudinal direction the electrons seems to be accelerated and then decelerated within the dipole. In the Lienard-Wiechert potential, the opposite sign in the longitudinal acceleration interfere destructively. At the edge of a dipole one of the term is missing, because either the electron is only decelerated (entering the dipole) or only accelerated (leaving the dipole) and the electron is emitting radiation. In this picture the analogy to transition radiation becomes apparent, because from the longitudinal point of view the electron seems to be strongly decelerated till it completely "stops", meaning that it is deflected out of the 1/gamma aperture of the acceptance angle of the observation.
The size of the fringe field is of significance for the observation of the edge radiation. If the electrons sees a long fringe field the longitudinal acceleration is suppressed, while the accumulated deflection angle pushed the radiation cone out of the acceptance angle of observation. The 'transition radiation' effect is strongly suppressed and the radiation looks more like the half of the sweeping cone of the synchrotron radiation.
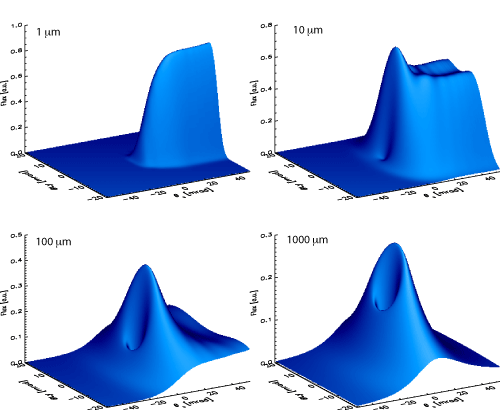
Fig.1: Edge radiation far field distribution for the ATF-Bunch compressor
Fig.1 shows the far field distribution of the edge radiation for the ATF bunch compressor at various wavelengths. At 1 micron the distribution is that of the synchrotron radiation, starting at 0 in the x-plane, with an tail of about 7 mrad into the negative half plane. Towards longer wavelength the distribution alters. The width becomes wider in the x-direction. At 1 mm the distribution is similar to that of the transition radiation, but with a slight asymmetry in x due to the residual part of the standard synchrotron radiation cone.
The explicit calculation of the radiation distribution is complicated, in particular if the observation point is not in the far field zone. In this case the contribution of the "velocity field" of the Lienard-Wichert potentials might be of importance as well. We refer to the report by R.A. Bosch, PAC Proceeding (1999) 2397 to solve the problem numerically.
Coherent Edge Radiation
The edge radiation is strongly enhanced, when the wavelength becomes comparable to or longer than the bunch length. Placing different high-pass filter in the radiation pass, allows to measure different integral parts of the radiation spectrum. From this information the bunch length and a possible strong modulation on a shorter length scale can be derived. E.g. measuring the spectrum between the third and forth dipole of a bunch compressor and then after the 4th dipole (using transition radiation) allows to obtain some information on the gain of the CSR microbunch instability, which mainly become visible in the 4th dipole and, thus, not seen by the edge radiation.
A significant problem for the coherence part of the edge radiation is the transverse beam size, which can be larger than the bunch length and thus increasing the wavelength, where the radiation is coherent. This might impose a limit on the bunch length measurement. Currently we are studying this effect by numerical simulation, using the Lienard-Wichert code TREDI.